This past Monday I recieved a surprise piece of mail from India. Once I got over my initial shock of getting a piece of personal mail, an event that has not happened in a long time, I quickly found that this piece of mail contained other surprises as well. First of all it was addressed to a Dr. Samuel Hansen, a title that I will accept but have in no way earned. My next surprise came when I opened it an found that it was a letter claiming that the author, a R. Sarva Jagannadha Reddy, had discovered the exact value of pi. This seemed to me to be a rather surprising claim, but I was willing to go along with it until I flipped the page and found that the claimed exact value of pi was $latex frac{14-sqrt{2}}{4}$ which equals 3.1464466…..
I found it somewhat improbable that the best mathematical minds in history had made a mistake on the thousandths digit of one of the most important and used constants in the world. So of course I did some quick googling to see if I could find out any more information about RSJ Reddy and, too my complete lack of surprise, I found out that I was not the first person to recieve this letter(apparently he will eventually send you a whole book about he value of pi). The one thing that I did not find was a complete takedown of the work. I am currently very busy working on Relatively Prime, and while crank baiting is fun it is not as important, so I was hoping that I could enlist the help of ACMEScience community for this project. Under this post I will include photos of every page of the letter and I want everyone to look through the mathematics and figure out where it goes off the rails and explain the where and how in the comment section below. Once all of the errors have been identified I will collect them together in a blog post. Remember this is a public service, when the next person receives this letter and goes to google, we want to make sure there is a clear explanation of just why it is complete, and utter, bug splat on the windshield of mathematics.
Have Fun!



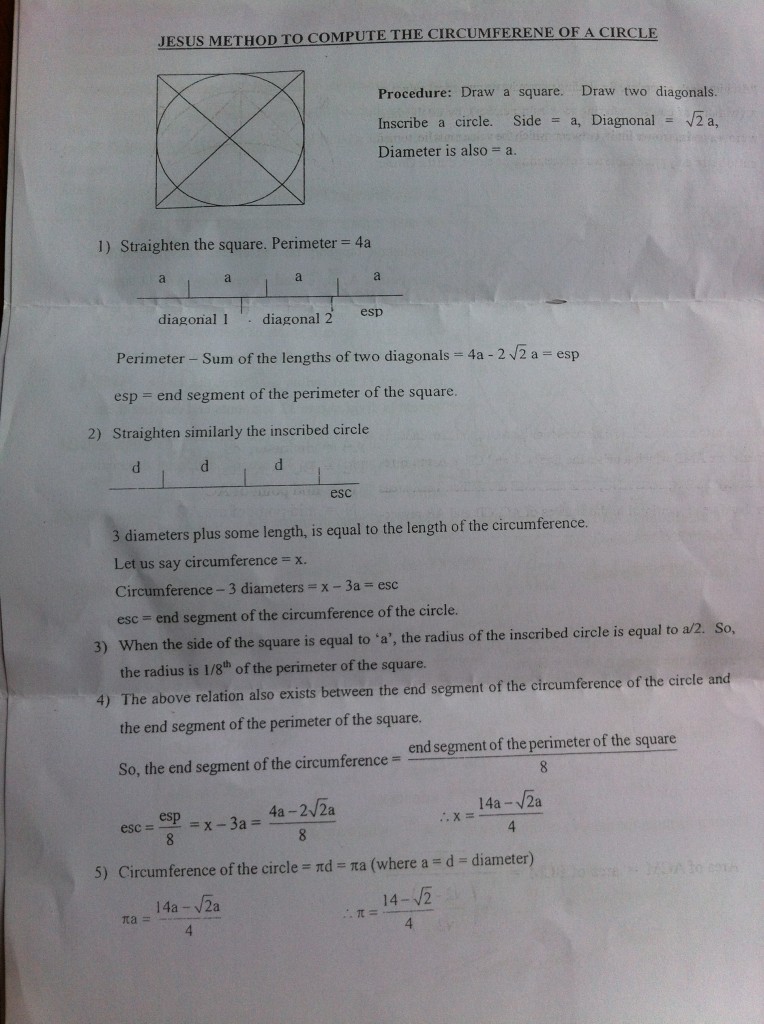


For the Jesus method, it has to be the not-so-obvious claim that esc = esp/8. However, I’m not sure how to disprove it.
How also did this man get my email address? The difference being that I know little about maths yet I have the most astounding “coincidences’ that anybody could imagine! I challenge ant of you to work me out!
Anybody email me rooney101015@gmail.com
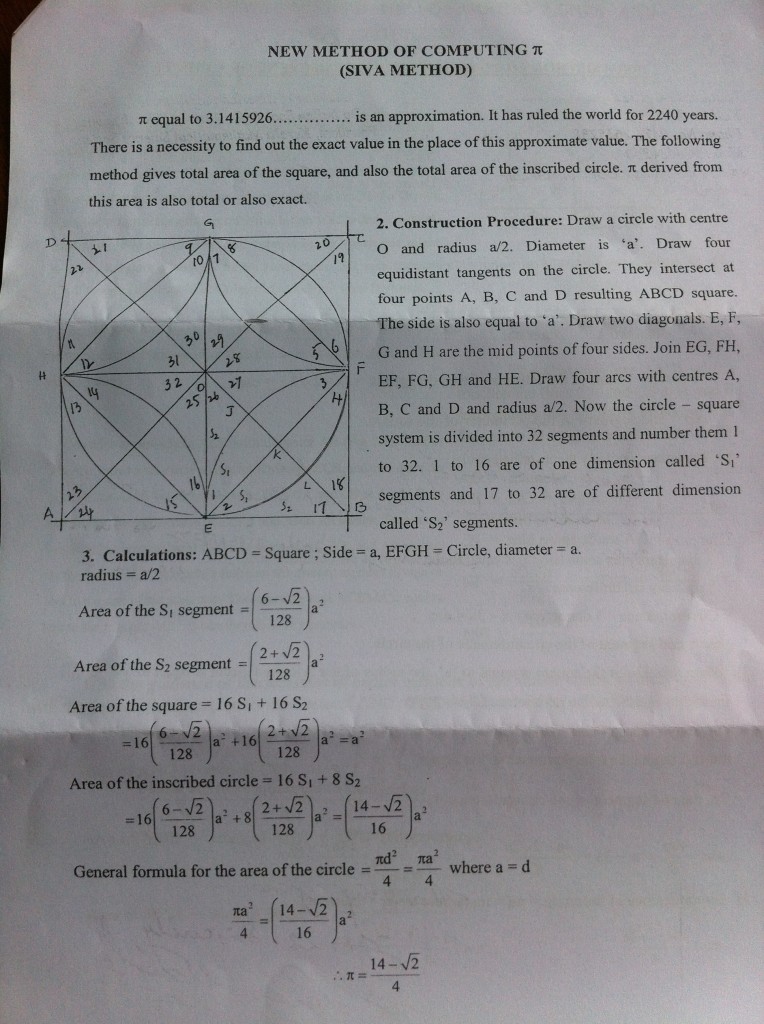
For the Siva Method, it’s where he states the areas of S1 and S2 segments, with those radical expressions. They can’t be right, but he doesn’t explain how he derived them.
The first computation contains “Area of the S1 segment” and “Area of the S2 segment,” with no explanation given for the formulae. The formulae are incorrect. (They’re algebraic multiples of d^2 and should be transcendental.)
In the second computation, “The above relation also exists Between the end segment of the circle and the end segment of the perimeter of the square.” What? No it doesn’t. (The argument is to take two “excess parts,” then assert that one is an integer multiple of the other, using an analogy that doesn’t hold.) This again ends up asserting that an algebraic quantity equals a transcendental quantity.
The third is a bit harder to follow, but I think the underlying error is to assume that if A+B=k and your formulae for A and B do add to give k, then your formulae for A and B are correct: “From the above equation, it is clear that the devised formulae for the areas of different segments is exactly correct.” There is of course plenty of sleight-of-hand with lunes and a scaling by root2 to obscure what’s going on.
For method 1, it’s the claim of what areas S1 and S2 are. I don’t have time to check what they should be atm, but the rest of that ‘proof’ doesn’t look nearly so suspect as just slipping that in like that.
For the Siva method, he’s using the answer he wants to get to (the value of pi) to give an incorrect calculation of the area of the S1 and S2 segments. Really, S1 has an area of (1/16)a^2(pi/2 – 1) and S2 has an area of (1/16)a^2-(1/16)a^2(pi/2 – 1)=(1/16)a^2(pi/2)=(1/32)pi*a^2.
The fact he doesn’t give his method for calculating these areas is an obviously sign of crankery (I used the well known formula you can find here: http://en.wikipedia.org/wiki/Circular_segment)
I think this is one error which is extrapolated through many apparent proofs.
BTW he is stating that 3.14159… is incorrect and that his value is actually correct. So it is not a problem, for him, that it gives a different value.
In the Jesus proof he is correct until he makes the remarkable intuitive leap that esc = esp/8. He provides absolutely no justification for this.
My guess is that this was his original proof and all the others rely on this one which is why they all give the same answer.
In the Siva method he is correct until he gives formulae for S1 and S2. No explanation is given but they happen to give the value of Pi he is looking for. So I presume they were calculated from the Jesus proof in some way.
The Hippocrates’ method is correct until he says ‘With the guidance of the formulae given by the author where a circle is inscribed with the square…’ So this proof is explicitly based on the Siva proof. The very next formula is incorrect.
It is easy to spot his incorrect formulae as they give algebraic values for transcendental numbers, specifically values involving Pi.
By the way maths is short for mathematics, no-one says mathematic. Surprised you haven’t got this point by now. Just saying.
Thanks for the great podcast and looking forward to relatively prime.
Cheers,
Steve
Siva: Agreed it’s the dropping out of the sky of the values for areas of S1 and S2 which are (pi-2)a^2/32 and (4-pi)a^2/32 with apologies (of the contradiction) to Daniel above. For the values that were given while their sum is correctly a^2/16 I’ve tried for the last day–in vain–to come up with a plausible way to incorrectly get the value for say the area of segment S1. This was quite surprising given that the relative error of S1 is only about 4.25%.
Jesus: As the other commenters have pointed out the error is in suggesting that the ratio in the defect of (4-2sqrt(2))a and (pi-3)a, esp/esc ~ 8.2742, corresponds to the to the ratio of the perimeter of a square with the radius of the inscribed circle which is exactly 8. There is no diagonal analogue like sqrt(2) for the circle and choosing 3 simply makes the defect less than one. Matthew’s explanation is quite good.
Hippocrates: In the first point the similarity of ANC, CPB with AMB only holds when the length DE = d/2 which is only spelled out in the caption of the figure. A quick computation shows that the area of AMB = (pi-2)a^2/2 and the area of ACBMA = (4-pi)a^2/2. Notice that these are the same values that show up in the Siva proof. The second point 1 seals our fate where the author states ‘with the guidance of formulae from earlier methods’ where the first expression for the area of segment AMB is tantamount to the replacement of pi by (14-sqrt(2))/4 which is the ‘not so’ surprising condition of the proof.
Probably that’s the way he’s advertising his books “The Circle”, “Pi of the Circle”(2008 – 326 pages), “New Method of Computing Pi” (2009 – 78 pages) and maybe more.
Any author of “Perpetuum Mobile” looks smarter than S.J.Readdy.
At least, they have some idea. But here the guy is trying to find exact value PI using approximation for PI. He could use approximation like PI =3. or 5. Although he mentioned Hippocrates & Pythagoras, it doesn’t make him intellectually closer to them.
I don’t buy his formulas for S1 and S2 in the author’s Sivi Method, which would both imply that the exact value of pi was known before determining it.
From the information given, it sounds like the author was just making up “complicated sounding” numbers to make his argument look good to those who do not know better.
sir,
my approach to the problem basically was to associate 3.1464466..exactly with that of the lenghts of the line segments of siva method, jesus method etc.
the derivation aspect of formule were immaterial to me due to my ignorance in mathematics( i am a student of zoology).
dr. gerry leversha , editor of the “mathematical gazette” (uk, 2003, july, page 368), has said ” …a trailblazing contribution to the canon…”
my work is in your hands and it can be disproved and NOT by comparing with the transcendental number 3.1415926..which is that of polygon.
rsj reddy
author
This is the only way to “square the circle,” http://www.circleissquared.com